It all started with a simple question: how do I calculate the area of a complex shape? As a budding math enthusiast, I found myself grappling with the limitations of traditional geometric formulas. That’s when I stumbled upon the magic of calculus. It felt like a whole new world of possibilities opened up, and at the heart of it all were these seemingly mysterious symbols: dx and dy. What exactly did they represent?

Image: www.reddit.com
Intrigued, I embarked on a journey to unravel the meaning behind “dx dy.” What I discovered was a fascinating exploration of infinitesimal change and its profound applications in various fields. In this article, we’ll delve deep into the world of dx dy, exploring its origins, its mathematical significance, and its far-reaching impact on our understanding of the universe.
dx dy: Unlocking the Secrets of Infinitesimal Change
At its core, “dx dy” represents the foundation of calculus, a branch of mathematics that deals with continuous change. Imagine a curve on a graph. How do you find the area beneath it? The answer lies in breaking the curve down into infinitesimally small rectangles, each with a width of dx and a height of dy. These infinitesimals, essentially infinitely small units, are the building blocks that allow us to calculate the area with remarkable accuracy.
To understand dx dy, we need to grasp the concept of derivatives. Imagine you’re driving a car and you want to know your instantaneous speed at a specific moment. The derivative gives you the rate of change of your position (distance traveled) with respect to time. Similarly, in calculus, the derivative of a function tells us how the function is changing at any given point, providing an instantaneous rate of change.
Exploring the Meaning of dx and dy
Let’s break down “dx dy” into its individual components:
- dx: This represents an infinitesimally small change in the independent variable “x.” In the context of our driving example, “x” represents time, and “dx” represents an infinitely small change in time.
- dy: This represents an infinitesimally small change in the dependent variable “y.” In our driving example, “y” represents distance, and “dy” represents an infinitely small change in distance.
Together, dx and dy represent a tiny rectangular area within the curve. By summing up all these infinitesimally small areas, we can approximate the total area under the curve. This technique, known as integration, is the inverse operation of differentiation. It allows us to calculate the total change of a function over a given interval.
The Significance of dx dy in Calculus
The notation “dx dy” is crucial in various areas of calculus, including:
- Integration: As mentioned earlier, dx dy plays a vital role in calculating the area under a curve using integration.
- Differentiation: While “dx dy” is most directly used in integration, understanding the concept of infinitesimal change is essential for comprehending derivatives. Without the notion of infinitesimally small changes, we wouldn’t be able to derive the instantaneous rate of change of a function.
- Multivariable Calculus: dx and dy extend their significance to higher-dimensional spaces, allowing us to analyze functions with multiple variables. The concept of partial derivatives, which measures the change of a multivariable function with respect to one variable while keeping the others constant, relies heavily on the principles of infinitesimal change represented by dx, dy, and their multivariable counterparts.
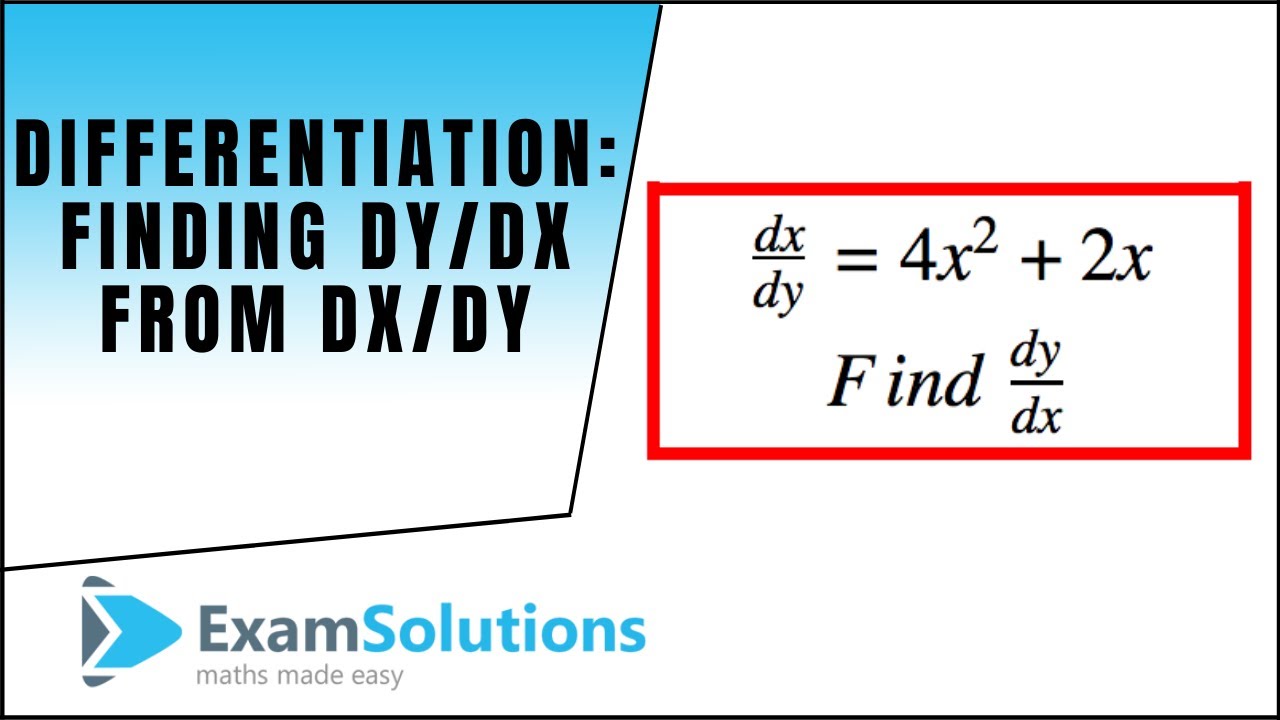
Image: www.youtube.com
The Impact of dx dy: Applications Across Disciplines
The principles of infinitesimal change embodied by dx dy are not merely theoretical constructs. They have profound practical applications in various fields, including:
- Physics: dx dy is crucial for understanding and modeling physical phenomena like motion, forces, and energy. For example, in calculating the work done by a force, we use the integral of “force × displacement,” which involves integrating infinitesimal changes in both force and displacement.
- Engineering: Engineers utilize calculus to design bridges, buildings, and machines, relying on the principles of infinitesimal change to analyze stresses, strains, and forces.
- Economics: dx dy plays a role in economic modeling, allowing economists to analyze changes in supply and demand, calculate marginal revenue and cost, and optimize production and consumption. For instance, the concept of marginal cost, which measures the change in total cost due to a one-unit increase in production, is a derivative concept based on the principles of infinitesimal change.
- Finance: dx dy is essential in quantitative finance, where it helps model financial markets, optimize portfolios, and understand the dynamics of stock prices.
The Power of Infinitesimal Change
The beauty of “dx dy” lies in its ability to represent change at its most fundamental level. It allows us to grapple with the continuous nature of our world and to quantify phenomena that would otherwise be impossible to measure. Through the intricate interplay of derivatives and integrals, we gain the power to analyze, predict, and manipulate the world around us with unprecedented precision.
Tips and Expert Advice for Embracing dx dy:
Embarking on the journey of understanding “dx dy” and its calculus applications might seem intimidating, but it’s an incredibly rewarding experience. Here are some tips to help you along the way:
- Start with the basics: Make sure you have a firm grasp of basic algebra and trigonometry before diving into calculus. A strong foundation in these areas will make your journey smoother.
- Don’t be afraid of limits: Calculus is all about limits – the idea of approaching a value without actually reaching it. Embrace this concept and practice working with limits to understand derivatives and integrals.
- Visualize the concepts: Draw graphs, diagrams, and visual representations to help you visualize the concepts of derivatives, integrals, and infinitesimals.
- Practice, practice, practice: Calculus is not a spectator sport! Practice solving problems, working through examples, and applying the concepts to real-world scenarios.
Remember, mastering calculus is like building a tower. Each concept builds upon the ones before it. Focus on understanding the underlying principles of “dx dy” and how they connect to the larger picture of calculus.
Frequently Asked Questions about dx dy:
Q: Is “dx dy” the same as “dy dx”?
A: No, “dx dy” and “dy dx” are distinct concepts. They are essentially flipped versions of each other, representing infinitesimal changes in different directions. In multivariable calculus, they play a crucial role in defining partial derivatives.
Q: Is “dx dy” always a rectangle?
A: While “dx dy” is often visualized as a rectangle, it doesn’t always have to be. In more complex scenarios, it can represent an infinitesimally small area of a different shape. The key concept is that it represents a change in both the x and y directions.
Q: What are some real-world examples of “dx dy”?
A: “dx dy” is used in countless real-world applications. For example, it’s used in calculating the volume of a container, in optimizing the efficiency of an engine, and in predicting the trajectory of a projectile.
Conclusion
Understanding the meaning of “dx dy” is a key step toward unlocking the power of calculus. It represents the foundation of infinitesimal change, a fundamental concept that permeates various branches of science, engineering, and economics. By embracing this notion of infinitesimal change, we gain the tools to analyze, model, and predict the behavior of complex systems.
Dx Dy Meaning
Are you intrigued by the world of dx dy and its applications?






