Ever wondered what a fraction like 17/20 would look like as a decimal? Perhaps you’ve encountered it in a math problem, or maybe you’re just curious about the relationship between fractions and decimals. Whatever the reason, the world of numbers holds many fascinating secrets, and the transformation of 17/20 into a decimal is one of them. This journey will take us through the basics of fractions, decimals, and how they intertwine, revealing the hidden value of 17/20 in the decimal system.

Image: brainly.com
Understanding the conversion of 17/20 to a decimal involves stepping into the world of fractions and decimals, two crucial mathematical concepts. Fractions represent parts of a whole, while decimals provide another way to express these parts, making them easier to work with in certain situations. This article will demystify the process of converting fractions to decimals, specifically focusing on the intriguing example of 17/20.
The Fundamental Fractions: A Building Block for Understanding
What are Fractions?
Fractions represent parts of a whole, much like slices of a pie. The top number, the numerator, tells us how many parts we have, while the bottom number, the denominator, indicates the total number of parts the whole is divided into. For instance, 1/2 represents one out of two equal parts of a whole.
Types of Fractions:
We encounter various types of fractions, each with its own unique properties.
- Proper Fractions: The numerator is smaller than the denominator, like 1/2 or 3/4. These fractions represent values less than one whole.
- Improper Fractions: The numerator is larger than or equal to the denominator, like 5/2 or 7/7. These fractions represent values greater than or equal to one whole.
- Mixed Numbers: These consist of a whole number and a proper fraction, like 1 1/2 or 2 3/4.
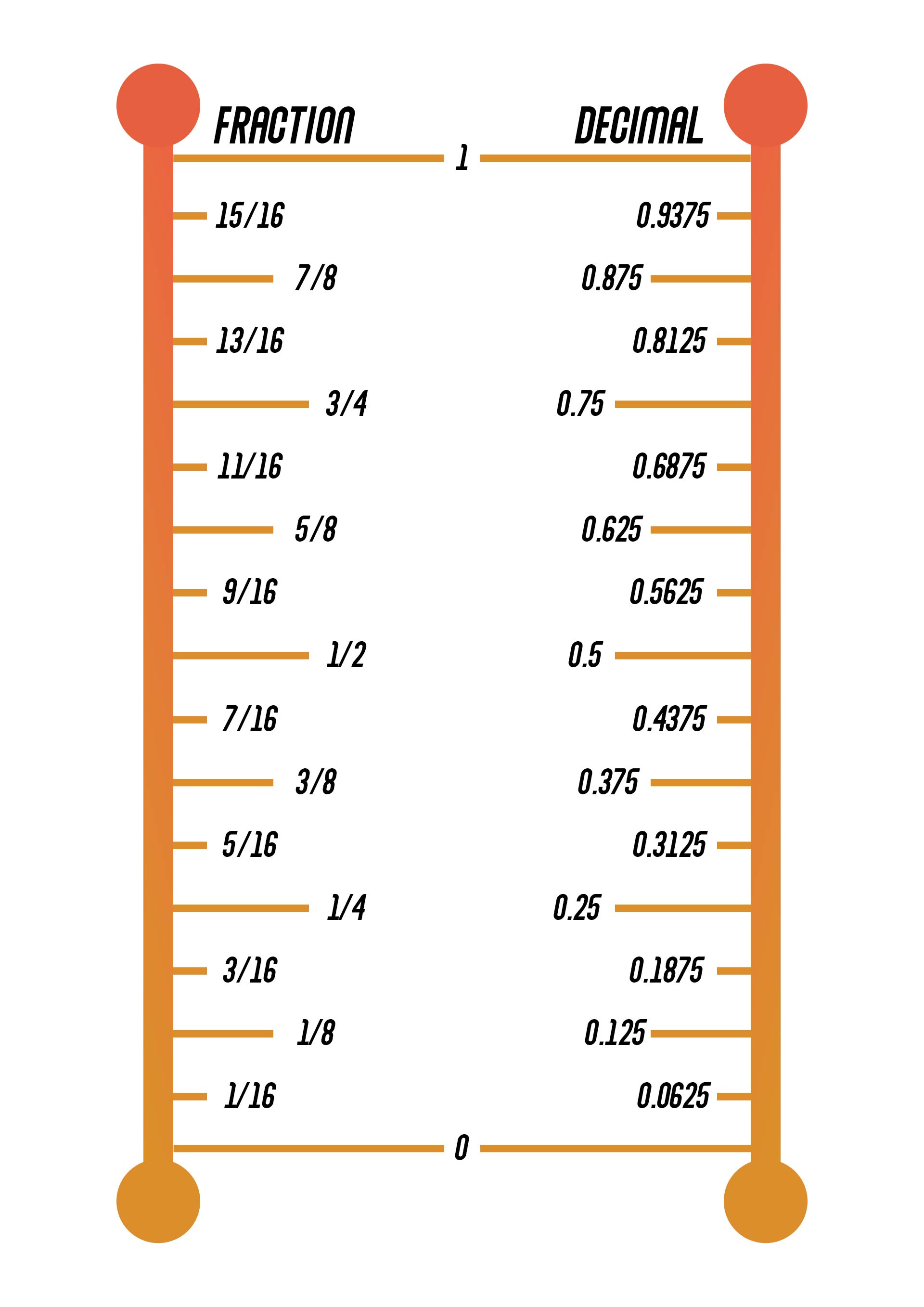
Image: printablecampusdorie.z21.web.core.windows.net
Unlocking the Decimal System: A Language of Tenths
What are Decimals?
Decimals provide a convenient way to represent fractions using the base-10 system. They are written using a decimal point (.), with each digit representing a power of ten. For example, 0.5 represents five-tenths, while 0.25 represents twenty-five hundredths.
The Significance of Place Values:
Understanding place values is crucial to working with decimals. Each digit’s position to the right of the decimal point indicates its value:
- Tenths Place: The first digit after the decimal point represents tenths, like 0.1 for one-tenth.
- Hundredths Place: The second digit represents hundredths, like 0.01 for one-hundredth.
- Thousandths Place: The third digit represents thousandths, like 0.001 for one-thousandth.
Converting Fractions to Decimals: A Step-by-Step Guide
Understanding the Method:
Converting a fraction to a decimal involves dividing the numerator by the denominator. The result obtained will represent the decimal form of the fraction.
Applying the Method to 17/20:
1. Divide the numerator (17) by the denominator (20). This gives us 17 ÷ 20.
2. Perform the division using long division or a calculator. The result we get is 0.85.
Therefore, the decimal equivalent of 17/20 is 0.85.
Real-World Applications of Decimal Conversion
Applications in Daily Life:
The conversion of fractions to decimals finds practical use in various aspects of our daily lives:
- Shopping: Discounts, sales, and price reductions are often expressed as fractions, like 1/2 off or 1/4 off. Converting these fractions to decimals helps us quickly calculate the actual discount amount.
- Cooking: Recipes frequently use fractions to indicate ingredient quantities. Translating these fractions to decimals makes it easier to measure ingredients accurately when using measuring cups or spoons.
- Construction: Construction projects involve working with dimensions and measurements, often expressed in fractions. Converting these fractions to decimals simplifies calculations and improves accuracy.
Applications in Science and Engineering:
Decimal conversions are indispensable in various scientific and engineering disciplines:
- Data Analysis: Scientists and engineers often work with data represented in fractional form. Converting these fractions to decimals facilitates data analysis, statistical calculations, and interpretation of results.
- Engineering Design: Engineering designs require precise measurements and calculations. Converting fractions to decimals enhances accuracy and eases the design process, especially when dealing with large figures.
- Financial Calculations: In fields like finance and accounting, decimals are employed for representing currency values, interest rates, and various financial transactions.
17/20 In Decimal
https://youtube.com/watch?v=EhgAyV3MNI4
Conclusion
Through this exploration of the decimal conversion of 17/20, we discovered that fractions and decimals are interconnected concepts, each providing distinct ways to represent parts of a whole. Understanding these concepts unlocks a world of mathematical possibilities, simplifying calculations and allowing us to navigate various aspects of our daily lives with greater ease. By embracing the power of fractions and decimals, we can approach mathematical challenges with confidence and unlock the secrets hidden within the numerical world.






