Have you ever felt overwhelmed by a physics problem, not because you didn’t understand the concepts, but because you were lost in a sea of units? I remember staring at a physics exam, feeling confident about the formulas, but struggling to align the units and arrive at the correct answer. That’s where dimensional analysis came to my rescue. It’s a powerful tool that can help you untangle complicated equations and ensure you’re working with the right units, simplifying the problem-solving process.
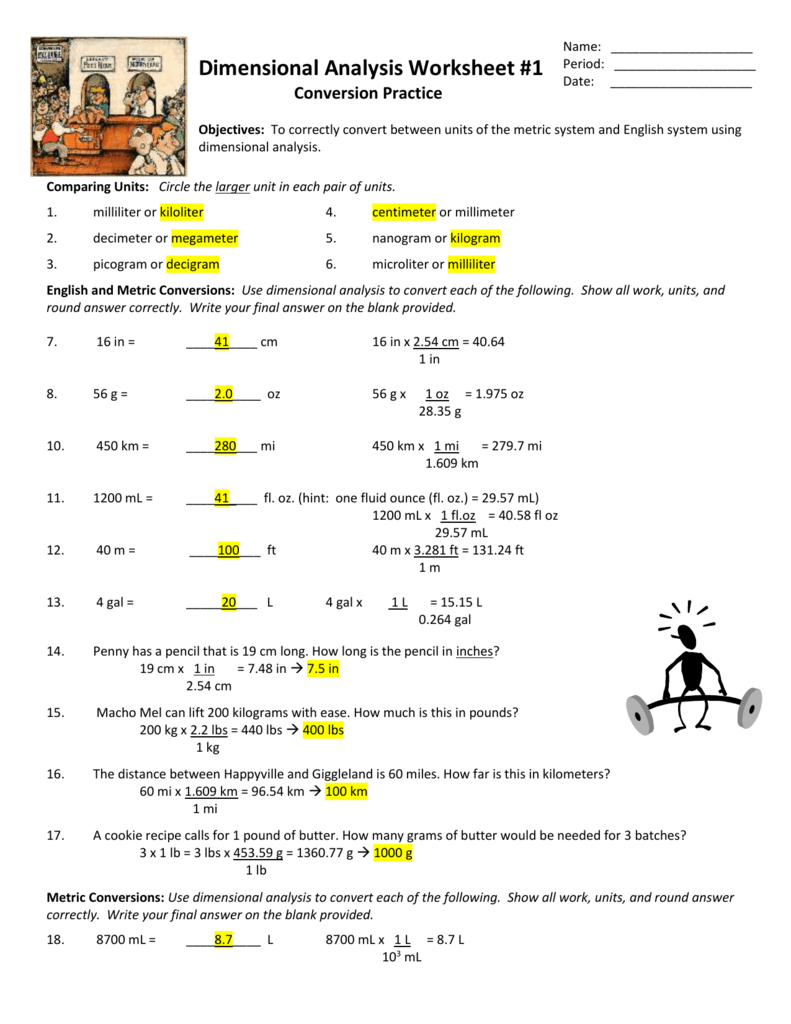
Image:
This article dives into the world of dimensional analysis, explaining its principles and how you can use it effectively. We’ll explore the concept, explore real-world applications, and guide you through creating your own dimensional analysis worksheets. Whether you’re a student grappling with physics, an engineer working on complex projects, or simply someone who wants to better understand the world around them, this article will provide you with the tools and insights to master the power of dimensional analysis.
Understanding Dimensional Analysis
At its core, dimensional analysis is a technique used to check the consistency of equations and ensure that the units on both sides of the equation match. It’s a powerful tool for verifying calculations, detecting errors, and understanding the relationships between different physical quantities.
The foundation of dimensional analysis lies in the concept of dimensions. Dimensions represent fundamental physical quantities, most commonly length, mass, and time. Each physical quantity, like velocity or force, can be expressed as a combination of these fundamental dimensions. For example, velocity is measured in meters per second (m/s), combining length (meter) and time (second).
The Power of Dimensional Analysis: How It Works
Dimensional analysis relies on the principle of dimensional homogeneity, where the dimensions of each term in an equation must be the same. This ensures that the equation is physically meaningful and that the units on both sides of the equation match. Here’s how dimensional analysis works in practice:
1. Identify the physical quantities involved
Start by clearly identifying the physical quantities involved in the problem. For example, if you’re calculating the volume of a cube, the quantities involved are length and volume.

Image:
2. Express each quantity in terms of its fundamental dimensions
Once you’ve identified the quantities, express each one in terms of its fundamental dimensions. Recall that length is represented by the symbol “L”, mass by “M”, and time by “T”. For instance, the volume of a cube (L^3) can be expressed as L*L*L, or simply L^3.
3. Substitute the dimensions into the equation
Substitute the dimensions of each quantity into the equation you’re working with. Remember to pay attention to the exponents of the dimensions. For example, if you’re calculating velocity (distance over time), you’ll substitute “L” for distance and “T” for time, resulting in L/T.
4. Check for dimensional homogeneity
After substituting the dimensions, check if both sides of the equation have the same dimensions. If they do, the equation is dimensionally consistent. If they don’t match, you’ve likely made an error in your calculations.
5. Analyze and resolve inconsistencies
If you discover inconsistencies in the dimensions, carefully examine the equation and try to pinpoint the source of the error. You might have missed a coefficient, used the wrong formula, or miscalculated the units of a particular term.
Real-World Applications of Dimensional Analysis
Dimensional analysis isn’t just a theoretical concept; it has practical applications in a wide range of fields:
- Engineering: Engineers use dimensional analysis to design and optimize structures, machines, and systems, ensuring that the units used in calculations are consistent and accurate.
- Physics: Physicists rely on dimensional analysis to formulate and test theories, verify experimental results, and gain a deeper understanding of physical phenomena.
- Chemistry: Chemists use dimensional analysis to convert between units, calculate quantities in chemical reactions, and ensure the consistency of measurements.
- Medicine: Medical professionals utilize dimensional analysis to correctly administer medication, interpret lab results, and ensure the accuracy of dosage calculations.
Creating Your Own Dimensional Analysis Worksheet
Dimensional analysis worksheets can be an excellent tool for practicing and solidifying your understanding of this technique.
1. Start with Simple Equations
Begin by creating worksheets with simple equations, focusing on basic units and concepts. For example, calculate the distance traveled at a given speed and time, or the area of a rectangle.
2. Gradually Increase Complexity
As you become more comfortable with dimensional analysis, gradually increase the complexity of the equations on your worksheets. Introduce equations involving multiple variables and units.
3. Provide Answers and Explanations
To make your worksheets more valuable, provide answers and detailed explanations for each problem. Explain how you arrived at the answer and highlight any common errors or misconceptions.
4. Focus on Real-World Scenarios
To make learning more engaging, create worksheets that reflect real-world scenarios. For example, calculate the fuel efficiency of a car or determine the volume of water in a swimming pool.
Expert Tips and Advice for Mastering Dimensional Analysis
Based on my experience and numerous successful applications of dimensional analysis, here are some expert tips to help you master this technique:
1. Focus on Understanding Units
Begin by developing a solid understanding of the units used in different physical quantities. Familiarize yourself with the standard units of measurement for length, mass, time, and other common quantities. Knowing the units will make performing dimensional analysis much easier.
2. Practice Regularly
Consistency is key to mastering any skill, and dimensional analysis is no exception. Regularly practice solving problems using dimensional analysis, starting with simple examples and gradually increasing the complexity. The more you practice, the more comfortable you will become with the concept.
3. Use a Step-by-Step Approach
When tackling complex problems, use a step-by-step approach to break down the problem into manageable parts. Start by identifying the quantities involved, expressing them in terms of their dimensions, and then substitute the dimensions into the equation. Follow this systematic method to minimize errors and ensure accuracy.
4. Don’t Be Afraid to Ask for Help
If you encounter any difficulties, don’t hesitate to ask for help! Search for resources online, consult textbooks, or reach out to a teacher, tutor, or mentor. Don’t be afraid to seek guidance and clarification from those who have experience with dimensional analysis.
Frequently Asked Questions About Dimensional Analysis
Q1: What is the difference between units and dimensions?
Units are specific measures for a physical quantity, while dimensions represent the fundamental quantities like length, mass, and time. For example, a meter is a unit of length, while length itself is a dimension.
Q2: Can I use dimensional analysis for all types of equations?
Dimensional analysis is applicable to equations that describe physical relationships and involve quantities with units. However, it might not be suitable for equations that purely represent mathematical concepts, where units are not relevant.
Q3: Is dimensional analysis helpful for solving problems?
Absolutely! Dimensional analysis is a valuable tool for solving problems involving units. It can help identify errors, verify calculations, and ensure that your results are consistent and physically meaningful.
Q4: How can I improve my skills in dimensional analysis?
Practice! Practice! Practice! The more you work with dimensional analysis problems, the more comfortable you’ll become with the process. Use worksheets, textbooks, and online resources to practice solving problems. Focus on understanding the concepts behind dimensional analysis, and don’t be afraid to ask for help if you need it.
Dimensional Analysis Worksheet
Conclusion
Dimensional analysis is a potent tool that simplifies solving problems involving units. It helps ensure the consistency of equations, verify calculations, and detect errors. By mastering dimensional analysis, you gain a deeper understanding of the relationship between physical quantities and their units. So, embrace dimensional analysis, practice regularly, and unlock the power of this fundamental concept in your study and work.
Are you interested in learning more about dimensional analysis, or do you have any specific questions you’d like answered?






