Imagine a chemist working in a lab, carefully adjusting the temperature of a reaction mixture. They observe that as the temperature rises, the reaction speeds up, leading to the production of more products. This observation, common in many chemical reactions, reflects the influence of temperature on chemical equilibrium. To quantify this relationship, scientists use a powerful tool called the Van’t Hoff equation.

Image: www.pinterest.ph
This equation, developed by the Dutch chemist Jacobus Henricus van ‘t Hoff, provides a mathematical framework for understanding how changes in temperature affect the equilibrium constant of a reversible reaction. The Van’t Hoff equation is crucial for predicting and controlling the outcome of chemical processes, impacting fields as diverse as pharmaceuticals, materials science, and environmental chemistry.
Delving into the Van’t Hoff Equation: Definition and Explanation
The Van’t Hoff equation expresses the relationship between the equilibrium constant (K) of a reaction and the absolute temperature (T), using the standard enthalpy change (ΔH°) of the reaction. It’s represented as:
The Equation:
d(lnK)/dT = ΔH°/RT²
Where:
- K: Equilibrium constant
- T: Absolute temperature (in Kelvin)
- ΔH°: Standard enthalpy change of the reaction (in Joules per mole)
- R: Ideal gas constant (8.314 J/mol·K)
This equation tells us that the change in the natural logarithm of the equilibrium constant with respect to temperature is directly proportional to the standard enthalpy change of the reaction, divided by the square of the absolute temperature and the ideal gas constant.
Understanding the Implications of the Van’t Hoff Equation
The Van’t Hoff equation holds significant implications for understanding and predicting chemical reactions. Let’s delve into some of the key takeaways:
- Endothermic vs. Exothermic Reactions: For endothermic reactions (ΔH° > 0), increasing temperature favors the formation of products. This is because the forward reaction absorbs heat, and raising the temperature provides more energy for the reaction to proceed. Conversely, for exothermic reactions (ΔH° < 0), increasing temperature favors the formation of reactants. As the forward reaction releases heat, raising the temperature shifts the equilibrium towards reactants to counterbalance the heat gain.
- Predicting Equilibrium Shifts: The Van’t Hoff equation allows us to predict the direction in which an equilibrium will shift when the temperature is changed. It can be used to determine whether a reaction will proceed further to the right (products) or to the left (reactants) when the temperature is increased or decreased.
- Optimizing Reaction Conditions: By understanding the effect of temperature on equilibrium, we can optimize reaction conditions for maximum product yield. This is especially important in industrial processes where maximizing efficiency is crucial.
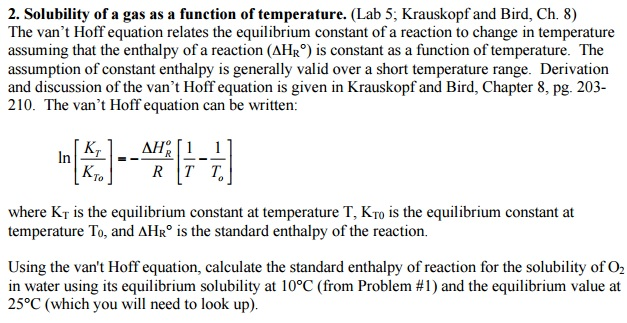
Image: www.chegg.com
Applications of the Van’t Hoff Equation
The Van’t Hoff equation finds applications in various scientific and technological fields:
- Pharmaceutical Industry: The equation plays a pivotal role in designing and optimizing drug synthesis processes, ensuring maximum production of effective pharmaceutical compounds.
- Material Science: By understanding the temperature dependence of equilibrium, scientists can predict and manipulate the formation of new materials with specific properties.
- Environmental Chemistry: The equation helps to model and predict the fate of pollutants in the environment, enabling more informed decisions for environmental protection.
Latest Trends and Developments
Recent advancements in computational chemistry are allowing researchers to generate more accurate and reliable predictions using the Van’t Hoff equation. These advancements involve incorporating complex quantum mechanical calculations and experimental data to refine the equation’s parameters and enhance its predictive power. For example, researchers are exploring new methods to incorporate the effects of non-ideal conditions, such as pressure and solvent effects, into the Van’t Hoff equation. This allows for a more comprehensive and nuanced understanding of equilibrium behavior in realistic chemical systems.
Tips and Expert Advice
Here are some tips for effectively using the Van’t Hoff equation:
- Units Matter: Ensure consistency in units for temperature, enthalpy change, and the ideal gas constant. Using Kelvin for temperature is crucial.
- Consider the Scope: While the Van’t Hoff equation is a powerful tool, remember that it applies to ideal conditions. It is important to consider potential deviations from ideality, such as non-ideal behavior of reactants and products or significant pressure changes.
- Embrace Experimentation: The Van’t Hoff equation provides a theoretical framework, but validating predictions through experimental verification is crucial.
Frequently Asked Questions (FAQs)
- Q: What is the significance of the standard enthalpy change (ΔH°) in the Van’t Hoff equation?
- Q: How do I apply the Van’t Hoff equation in real-world scenarios?
- Q: What are some limitations of the Van’t Hoff equation?
A: ΔH° represents the heat absorbed or released during the reaction under standard conditions. Its sign indicates whether the reaction is endothermic (heat absorbed, positive ΔH°) or exothermic (heat released, negative ΔH°). The magnitude of ΔH° determines how strongly temperature affects the equilibrium constant.
A: In practical settings, the Van’t Hoff equation is often used in conjunction with experimental data. You can measure the equilibrium constant at different temperatures and use the Van’t Hoff equation to determine the standard enthalpy change of the reaction. This knowledge can then be used to predict equilibrium behavior at other temperatures or optimize reaction conditions for desired outcomes.
A: The Van’t Hoff equation is based on the assumption of ideal behavior, meaning that it applies only to reactions in ideal conditions. However, in reality, reactions may deviate from ideality due to factors like pressure changes, non-ideal behavior of reactants and products, and solvent effects. Therefore, the Van’t Hoff equation may not always provide perfectly accurate predictions in practical scenarios. It’s important to consider these limitations and adjust the equation’s parameters accordingly for more reliable predictions.
Vant Hoff Equation
Conclusion
The Van’t Hoff equation provides a powerful framework for understanding the relationship between temperature and equilibrium in chemical reactions. It has broad applications in scientific fields, impacting drug discovery, materials development, and environmental monitoring. Using this equation, scientists can predict the direction of equilibrium shifts in response to temperature changes, optimize reaction conditions for maximum yield, and model complex chemical processes. While the Van’t Hoff equation is a valuable tool, it’s important to remember its limitations and incorporate experimental data to refine its predictions.
Are you interested in exploring the Van’t Hoff equation further? If so, let’s continue the discussion in the comments below.






