Have you ever stared at a busy street intersection, with lines of cars crossing each other at all angles, and wondered if there’s actually a hidden order beneath the apparent chaos? Perhaps you’ve gazed at a railroad track stretching endlessly into the horizon, realizing that the parallel lines never intersect. These everyday observations are actually a gateway to understanding the fascinating world of geometry. Today, we’ll delve deeper into the fascinating world of parallel lines and transversals. This not just an academic pursuit, but a window into the way our world is structured.

Image: materialdbwerfel.z19.web.core.windows.net
Imagine yourself as a detective. You’re looking at a crime scene, carefully inspecting the clues. Suddenly, you notice a set of footprints – a clue that might reveal the movements of the perpetrator. But these footprints are not just any footprints. They’re parallel, indicating the suspect moved in a consistent direction, offering crucial insights into their path. Just like a detective utilizes clues to unravel a mystery, we’ll explore how parallel lines and transversals provide valuable insights into geometric relationships, helping unravel the intricate patterns of our world.
The Fundamentals: Parallel Lines, Transversals, and Their Dance
Let’s begin with the basics. Parallel lines, as the name suggests, are lines that run side-by-side and never intersect, no matter how far they extend. Imagine two straight roads running alongside each other – they may appear to get closer together in the distance, but they will never actually meet. Now envision a third line that cuts across both of these parallel lines – that’s your transversal. It’s like a crossroad connecting two parallel roads, forming a series of angles with each of the lines.
The relationship between parallel lines and transversals is a symphony of angles. The angles created when a transversal intersects parallel lines exhibit specific patterns and relationships. Understanding these relationships is essential for solving geometric problems, whether it’s calculating the size of an unknown angle or proving a theorem.
Exploring the Angle Relationships: A Guided Tour
When a transversal cuts through parallel lines, a series of eight angles are formed. These angles are not just any random angles; they’re related to each other in predictable and harmonious ways. Let’s explore these relationships:
1. Corresponding Angles: Mirror Images
Corresponding angles are like mirror images of each other. They occupy the same relative position at each intersection. Imagine one angle as your reflection in a mirror – its corresponding angle is like your actual self, identical in size and shape. These angles are always congruent, meaning they have the same measure.
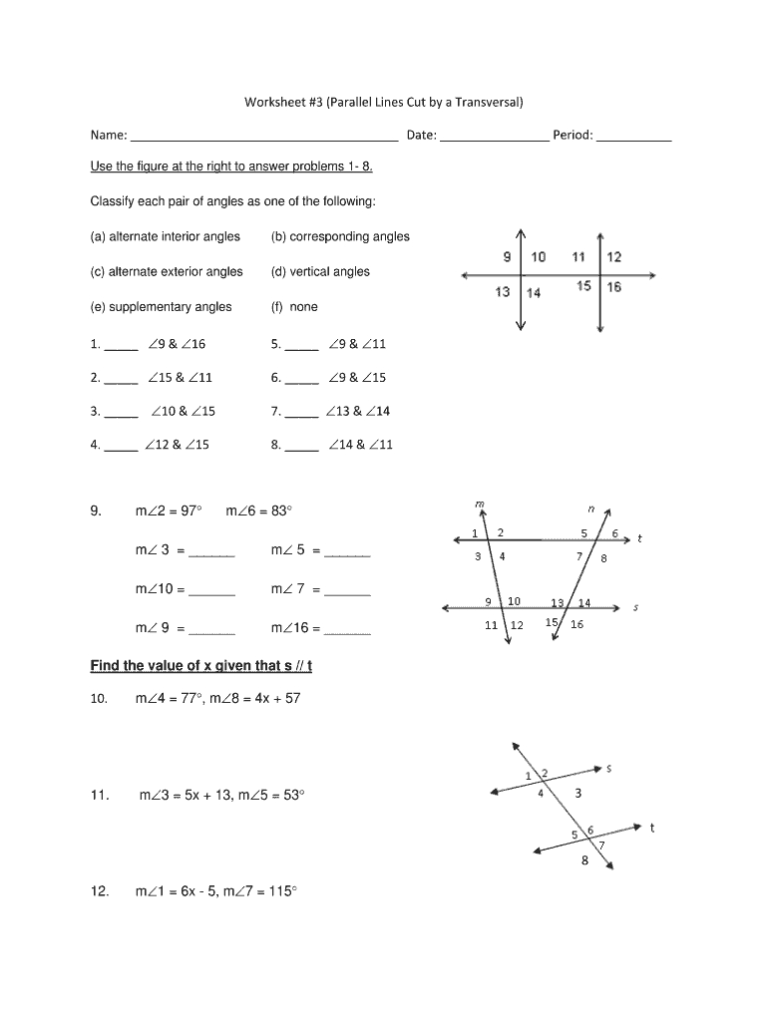
Image: quizzschoolplumpness.z5.web.core.windows.net
2. Alternate Interior Angles: Dance Partners
Alternate interior angles are found on opposite sides of the transversal, nestled between the parallel lines. They’re like two dancers performing a synchronized routine, always moving in harmony. Alternate interior angles are also congruent – they have the same measure.
3. Alternate Exterior Angles: The Opposite Sides
Imagine the transversal as a dividing line, and you’re standing on one side. The alternate exterior angles are located on the opposite side of the transversal, outside the parallel lines. This pair of angles also share the same measure – they are congruent.
4. Same-Side Interior Angles: A Complementary Duo
These angles are located on the same side of the transversal, between the parallel lines. Unlike the congruent pairs we’ve explored so far, these angles are supplementary. This means their measures add up to 180 degrees – like two puzzle pieces fitting perfectly together.
5. Same-Side Exterior Angles: A Similar Pairing
These angles are located outside the parallel lines and on the same side of the transversal. Like the same-side interior angles, these angles are also supplementary – their measures add up to 180 degrees.
The Parallel Lines and Transversals Worksheet: Your Pathway to Mastery
These worksheets are your secret weapon, providing practice problems designed to solidify your understanding of parallel lines and transversals. Engaging with these worksheets is like participating in a geometric game! Each problem presents a specific scenario involving parallel lines and a transversal, challenging you to apply the angle relationships we’ve discussed.
Types of Problems: A Variety of Puzzles
You might be asked to calculate the measure of a missing angle using the angle relationships. Alternatively, you might be tasked with proving that a pair of lines are parallel given a set of angles. Or, you might even face scenarios where you need to use multiple angle relationships simultaneously to solve a problem.
Benefits of Practice: Nurturing Your Geometric Skills
The benefits of working through these worksheets are numerous. It helps you develop a deeper understanding of parallel lines and transversals, strengthens your problem-solving skills, and equips you with the confidence to tackle more complex geometric challenges. Each solved problem becomes a building block, paving the way to a stronger foundation in geometry.
Expert Insights: Mastering Parallel Lines and Transversals
Here’s what prominent educators and mathematicians say about mastering parallel lines and transversals:
-
Dr. Maya Santos, Professor of Mathematics, University of California, Berkeley: “Understanding parallel lines and transversals isn’t just about memorizing a bunch of rules – it’s about grasping the underlying geometric relationships. When you see those angles, you should visualize the connections and relationships, not just a collection of numbers.”
-
Dr. Thomas Chen, Author of “Geometry Made Easy”: “Think of parallel lines and transversals as a language that helps you communicate about geometric shapes. Once you master this language, you can express yourself effectively and solve complex problems with ease.”
Actionable Tips: Unleashing Your Geometric Potential
Here are some practical tips to maximize your learning with parallel lines and transversals worksheets.
-
Visualize the Relationships: Don’t just read the problem text – visualize the scenario. Draw a diagram to represent the parallel lines, transversal, and angles. This visual aid will help you comprehend the relationships much more effectively.
-
Use Highlighting or Color-Coding: Highlight or color-code corresponding, alternate interior, or alternate exterior angles to clearly identify the relationships.
-
Practice, Practice, Practice: The more you practice, the more comfortable you’ll become with applying the angle relationships. The worksheets are your tools for building this confidence.
-
Seek Clarification: If you encounter a problem you’re unsure about, don’t hesitate to ask for help. Reach out to your teacher, a tutor, or search for online resources for additional explanation.
Parallel Lines And Transversals Worksheet
Conclusion: Entering a World of Geometric Harmony
We’ve embarked on a journey exploring the fascinating world of parallel lines and transversals. We’ve discovered the fundamental concepts, delved into the intricate relationships between angles, and explored the benefits of mastering this topic through practice worksheets. Remember, geometry is not just about abstract shapes – it’s about the patterns and relationships that govern our world. By mastering parallel lines and transversals, you unlock a new understanding of the beauty and order that exists within our surroundings.
Now, it’s your turn to delve into this world. Grab your parallel lines and transversals worksheet, unleash your geometric potential, and enjoy the journey of discovery!






